How many audio channels do digital organs need?
Colin
Pykett
Posted:
4 July 2017
Revised:
4 July 2017
Copyright
© C E Pykett 2017
Abstract.
Digital organs are well known for their tendency to fatigue the ear when played loudly with many stops drawn. This commonly happens even in instruments which sound well
with quieter combinations. The phenomenon is partly due to intermodulation distortion arising mainly in the loudspeakers which causes very large numbers of spurious sum and difference tones to be added to the sound. It is quite possible for there to be hundreds of thousands of distortion products, and although each is of low
amplitude their sheer number causes an audible background of acoustic mush to arise in the radiated sound. As a result the sound loses transparency and it becomes wearisome and identifiably electronic. Pipe organs do not suffer from this defect at all.
One way to eliminate the problem is to use one or more banks of twelve independent audio channels, each handling only one note and its octaves. There is then no opportunity for intermodulation to occur. However the number of amplifiers and loudspeakers required can become excessive when several such banks are required, and this article
shows how it can be reduced up to threefold using banks of no more than four channels. This simplification is possible because it is
unnecessary to use separate channels for the notes comprising dissonant intervals. Their subjective coarseness in effect masks the distortion products which they generate.
This approach cannot be applied to the purer-sounding consonant intervals however, which must therefore retain separate channels for their respective notes. This is essential if all the major and minor triads are also to be radiated without intermodulation distortion, because each triad is built from consonant intervals. As triads form the foundation of all harmony, it is important to handle them properly in a low-distortion channel assignment scheme.
The article shows how to assign notes to banks of six and four audio channels to enable these principles to be applied.
Both configurations represent a considerable saving in terms of hardware and cost over that of a twelve-channel system.
Contents
(click
on the headings below to access the desired section)
Introduction
Consonant
and dissonant intervals
Theories
of consonance and dissonance
Audio
system design - channelisation
Examples
of channelisation
12-channel
system
6-channel
system
4-channel
system
Concluding
remarks
Notes
and References
Introduction
In 2005 I wrote an article [1] which has become one of the most-quoted and controversial on this website. Today (2017) it still continues to arouse discussion if not passion about several of the issues raised, though this might be partly generational because on this timescale a new generation of enthusiasts is now probably entering the fray. It is certainly the case that the old questions seem to be arising again twelve years on.
One question concerns the number of independent audio channels required for digital organs or virtual pipe organs. Although there is widespread acceptance that instruments with more channels will usually sound better than those with fewer, the technical justification for this belief is less well understood and this is probably because it involves some rather arcane issues. Two of these were explored in detail in the earlier article. One concerns intermodulation (IM) distortion and the other concerns phase interference caused by mixing multiple signals electronically rather than allowing them to mix acoustically in the listening room. This article now takes the discussion further, mainly relating to IM distortion though it has relevance to the signal mixing problem also.
It is routine practice for the note signals associated with a single stop to be mixed into a common channel before being fed to an amplifier and then a loudspeaker system (there might be two channels if the stop is radiated in stereo but this does not affect the following arguments). This means that many frequencies are forced down this channel, the exact number depending on how many notes are keyed simultaneously multiplied by the number of harmonics associated with each one. This can potentially be a large number. For instance, if a chord of eight notes is played on a loud reed with perhaps 50 harmonics per note, then the channel is being asked to cope with around 400 separate frequencies. If there is IM distortion in the channel, and there always is (with most of it being contributed by the loudspeaker), then
well over 300,000 sum and difference frequencies will be generated - you can do the maths yourself if you like but it is the final answer which matters! The amplitude of each of these distortion products is small in isolation (distortion figures for good loudspeakers are typically around 0.5%), but when there are so many of them they combine to produce an audible background of acoustic mush which considerably degrades the listening experience. This is the principal reason why a digital organ, which might sound well with just one or two stops in use, will often be tiring and perhaps unpleasant when played loudly. The problem gets even worse when more stops are added to the ensemble.
A solution described in the earlier article was to route all twelve notes and their octaves through twelve different channels, virtually eliminating IM distortion and leaving only harmonic distortion. Although this also can be audible, it is less objectionable and easier on the ear because its distortion products are at frequencies already present in the input signals. In contrast, the majority of the distortion products in IM distortion lie at frequencies not in the input. Because there are so many of them, IM distortion appears as a quasi-continuum of gritty-sounding acoustic noise across the entire audio spectrum, radiated into the room along with the desired signals. The pipe organ is free of such problems of course.
The problem with the 12-channel scheme is the number of audio channels required, particularly for large instruments where several or many
banks of channels could be called for. Therefore this is addressed in the present article, which shows that six or even four channels are almost as effective as twelve as far as suppressing IM distortion is concerned. This is perhaps a surprising result. The main reason for the simplification derives from the fact that a 12-channel system treats all intervals as equivalent in terms of how we perceive them. Thus the notes forming the purest consonant interval (an octave) are treated in the same way as those forming the coarsest dissonant one (a minor second) in that the notes of both are assigned to independent audio channels. However it is unnecessary to do this when it is realised that the subjective coarseness of a dissonant interval can be harnessed to advantage. By sending its two notes through the same channel, the harsh sound of the interval will tend to mask the low level IM distortion which the notes generate. This cannot be done with the purer-sounding consonant intervals, all of which must use independent channels for their constituent notes. This is necessary so that the building blocks of harmony, the
twelve major and twelve minor triads all constructed from consonant intervals, can also be radiated through separate channels without allowing IM distortion to arise.
Using this simplification, the article shows that even a 4-channel system can offer nearly all the advantages of a fully separated 12-channel one in terms of reducing IM distortion: the notes of all 72 consonant intervals and all 24 triads are routed separately, and of the 60 dissonant intervals only 24 use the same channel. IM distortion will arise for these 24 intervals, but since they are dissonant it is doubtful whether it will be subjectively important. This is a modest price to pay for the
significant economies gained by using only one third the number of amplifiers and loudspeakers of a fully separated 12-channel system.
Consonant and dissonant intervals
We first need to consider the musical intervals which arise when two notes on a keyboard instrument sound simultaneously as in harmony rather than successively as in melody. Such intervals are said to be either consonant or dissonant, though the definitions of these terms have been disputed for centuries and it is not the intention to get bogged down in this particular morass. Here we are considering physics and audio engineering rather than musicology. Thus it is sufficient to regard consonant intervals as those which sound pleasant and musically 'resolved', whereas dissonant intervals are those which do not. An example of a consonant interval is that formed by a note and its octave, and of a dissonant interval a minor 2nd (the interval formed by two adjacent notes separated by a semitone). However these are the extremes of maximum purity and maximum harshness in keyboard instruments, and the majority of intervals fall into the somewhat ill-defined perceptual region between them. The problem of where to draw the distinction within this grey area is responsible for the divergence of academic opinion which still continues.
Nonetheless, there is broad (though not universal) agreement that the following intervals are consonant:
Unison, Octave, Perfect Fifth, Perfect Fourth, Major Third, Minor Third, Major Sixth, Minor Sixth.
If we ignore the first two for now as being less important to the discussion, this means there are six consonant intervals to the octave for each starting note. Since there are twelve possible starting notes (A, A#, B ... G#), there are therefore 72 consonant intervals to each octave across the key compass (excluding the unisons and octaves).
Thus the remaining intervals are dissonant:
Major Second, Minor Second, Augmented Fourth, Major Seventh, Minor Seventh.
The Major Second and Minor Second are the intervals formed by notes a whole tone (e.g. C-D) and a semitone
(e.g C-C#) apart respectively. The Augmented Fourth (e.g. C-F#) is not distinguishable on a keyboard from a Diminished Fifth with the same root note
(C-G♭) because the physical notes played are the same. Therefore the latter, although an interval with a separate musical existence, is not included here. Thus there are five dissonant intervals to the octave for each starting note. Since there are twelve possible starting notes, there are 60 dissonant intervals to each octave across the keyboard.
Theories of consonance and dissonance
Theories of consonance and dissonance have come and gone since time immemorial. In the nineteenth century Helmholtz proposed a theory which was initially based largely on the physics of musical sounds, although he later moved beyond this position of happy simplicity owing to pressure from the musical fraternity. Nevertheless, his simple model is sufficient for this article whose focus is more on the physics rather than the music itself, and in particular on how to radiate musical sounds optimally from a loudspeaker array. Reduced to their essentials, Helmholtz's arguments were based on the fact that the notes forming a consonant interval possess certain harmonics which coincide exactly in frequency when the interval is perfectly tuned. For instance, in a perfect fifth the third harmonic of the lower note has the same frequency as the second harmonic of the upper note (together with other similar correspondences of higher harmonics). In fact the practical definition of a perfectly-tuned interval is that it exhibits no beats between any of its coincident harmonics. In practice most consonant intervals cannot be tuned exactly because of restrictions imposed by the temperament used - in equal temperament for example, only the octaves are perfectly in tune. However the other slightly-out-of-tune consonant intervals are nevertheless broadly tolerable because their harmonics only generate relatively slow beats. In contrast, the harmonics in dissonant intervals do not even come close to overlapping in frequency when the notes are properly tuned within a temperament. This results in a dense jungle of pronounced beats which can produce a jarring aural effect verging on the aggressive or unpleasant. While this brief description skates over the complexity of the subject, hopefully it should express its essentials.
Audio system design - channelisation
Helmholtz's distinction between the two types of interval based on the beat frequencies between their harmonics is pivotal to the arguments in this article. It is suggested that the subjective cacophony of dissonant intervals can be harnessed to perceptually mask the shortcomings of audio systems, in particular intermodulation (IM) distortion. All real audio channels are sufficiently nonlinear to generate audible IM distortion products when there many of them, with the nonlinearities arising mainly in the loudspeakers.
Nonlinearity results in a perceptible quasi-continuum of acoustic background mush due to a huge number of low level sum and difference tones distributed across the audio spectrum, because it transfers signal power into distortion products at all the beat frequencies. (There is actually no power at a beat frequency so in an ideal, perfectly linear, channel the beat will not exist as a separate tone). However, because of the relatively harsh nature of dissonant intervals it is suggested that their notes can be
allowed to mix and be handled within a single audio channel with its intrinsic nonlinearity without significantly increasing the perceived aural degradation. The proposal relies on one psycho-acoustic percept (the subjectively coarse nature of dissonant intervals) masking another (the background due to IM distortion).
On the other hand, the notes comprising the purer sound of a consonant interval must always be routed through separate channels and loudspeakers to avoid generating audible IM distortion. If a single channel were used, distortion products would result partly from the small frequency differences which necessarily arise when perfect intervals are deliberately detuned to accommodate a particular temperament. This happens with all temperaments to some degree or other. However another major source of distortion products exists even with perfectly tuned consonant intervals, an example being the perfect fifth which will generate a tone one octave below the lower note if IM distortion is present. This suboctave frequency equals the difference between the two fundamental frequencies. Many other sum and difference tones will also be generated.
The octaves are the only exception to this separate-channel rule for the notes of consonant intervals because they are always perfectly tuned, or very nearly so. Therefore small frequency differences between corresponding harmonics do not generate IM distortion products, simply because there are no such differences. In addition, all harmonics of the lower note (other than the fundamental) overlap exactly with those of the upper, and this attribute makes the octave unique among the consonant intervals. Doing the arithmetic will therefore show that the frequencies of all the sum and difference products coincide with those already existing in the constituent notes, which means that IM distortion as generally understood does not exist. IM distortion only occurs when power is created at frequencies not present in the original signals. Instead, the interval of an octave passing through a nonlinear audio channel generates only harmonic distortion. This is less disagreeable to the ear than IM distortion because the frequencies of the distortion products coincide with those already present in the individual notes. Therefore, and unlike the other consonant intervals, in practice it is not objectionable to route octaves through a single channel. The same also applies to unisons.
The fundamental task is therefore to design an audio system which will always route the notes of all consonant intervals (other than octaves and unisons) through different amplifiers and loudspeakers to avoid IM distortion. An equally important task is likewise to route the notes of all the major and minor triads through different audio channels, because these triads form the basis of harmony in Western (and therefore organ) music. There are twelve major and twelve minor triads to the octave. Although this second requirement might seem forbiddingly difficult at first sight, in fact it will be met automatically when the first has been satisfied. Here's why. A major triad consists of a minor third sitting on top of a major third. Taking the major triad C-E-G as an example, the lower interval C-E is a major third and the upper interval E-G a minor third. But in a minor triad the two thirds are reversed - it consists of a major third sitting on top of a minor third. In the minor triad
C-E♭-G, the lower interval C-E♭ is now the minor third whereas E♭-G is the major one. Also, in both major and minor triads the bounding notes (C and G in this example) form a perfect fifth. Therefore three intervals are involved in any triad - major and minor thirds and a perfect fifth. Because all are consonant, the constituent notes of all 24 triads will always be routed through independent audio channels provided this happens for all of the 72 consonant intervals within the octave. And if there is sufficient flexibility in the design, it will also be an attractive spinoff for as many dissonant intervals as possible to be routed through different channels as well as all the consonant ones. This is because the dissonant intervals have subjectively different degrees of dissonance - they are not all as subjectively 'bad' as the interval of a semitone. Therefore it will obviously be advantageous if a channelisation scheme routes at least some of them via different channels.
Examples of channelisation
12-channel system
This is the scheme suggested in the earlier article [1] in which all twelve
notes (A, A#, B ... G#)
and their octaves are routed via separate audio channels, each channel having its own amplifier and loudspeaker system. This could be realised by providing twelve channels for the whole instrument, or each division (swell, great, etc) might have its own set of twelve. In the most elaborate implementations small groups of stops or even single stops might have their own 12-channel array. The latter arrangements would then also address the problem of phase interference due to electronic (rather than acoustic) signal mixing which was fully discussed in the earlier article. The advantage of even the least elaborate system with just one set of twelve channels is that IM distortion would be eliminated, because the notes comprising all intervals, both consonant and dissonant, would be routed through independent channels (except for the octaves and unisons where IM distortion is not an issue). The obvious disadvantage is the number of channels required, and it is this aspect which the present article addresses by suggesting that the notes of at least some of the dissonant intervals could share the same channel in a reduced scheme.
6-channel system
In this system, groups of two notes formed from adjacent semitones share a common audio channel. Thus C and C# could be one group, D and D# the next and so on, though it is immaterial how the groups are arranged. For instance, B and C could form one group, C# and D the next, and so on. It is only necessary that pairs of adjacent semitones are grouped. Irrespective of the group configuration, six such groups will therefore cover an octave rather than the twelve of the previous scheme. Consequently the number of amplifiers and loudspeakers required is halved, which is obviously a major advantage. As before, the whole instrument could use a single array of six channels, or there might be separate 6-channel arrays for each division, for small groups of stops or even for individual stops. Also as before, the more elaborate realisations would address the problem of phase interference due to electronic signal mixing.
It is immediately apparent that twelve of the most objectionable dissonant intervals, those formed by adjacent notes, are each handled by a single audio channel in this scheme, and the justification for this was discussed earlier. It relies on the coarseness of dissonant intervals masking intermodulation distortion in the channel. However it is necessary to analyse in more detail the assignment of intervals to audio channels in this system before drawing further conclusions. Let us therefore look at a system with the following channel assignments:
Channel 1: C and C#
Channel 2: D and D#
Channel 3: E and F
Channel 4: F# and G
Channel 5: G# and A
Channel 6: A# and B
The way that the various notes and intervals are distributed across the six audio channels is shown in Table
1 below:
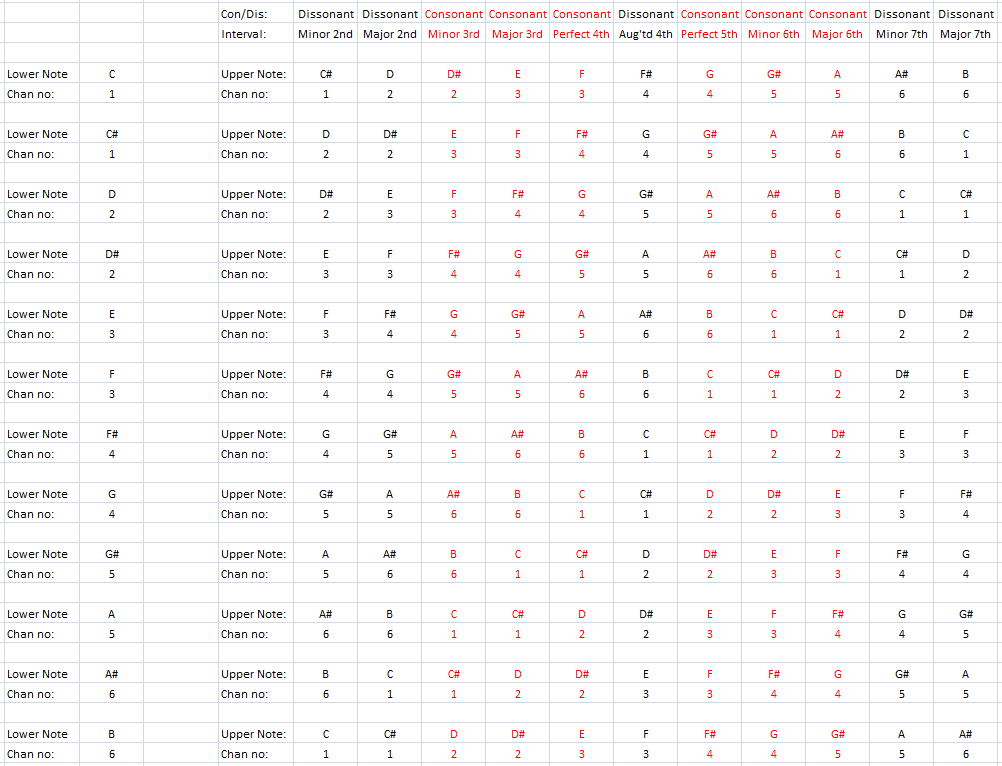
Table 1. Distribution of notes and intervals to audio channels - 6 channel system
At first sight this is a complex chart and it will probably take time to become familiar with it. In the top left corner is written 'Lower Note C'. This means that, first of all, we will examine all of the eleven intervals whose lower note is C
(i.e. omitting the octave). We come to the first one by moving along the row to the right. This is formed with the upper note C#, and the headings at the top of the column inform us that this interval is both dissonant and a minor 2nd. It is formed by two adjacent notes which, as seen already, share audio channel 1. Throughout this table the channel numbers appear underneath each note.
Continuing to move along the same row, we come to the next interval which is the dissonant major 2nd formed with the upper note D. This time the two notes are assigned to separate channels even though this interval is also dissonant.
Proceeding in the same way brings us next to the minor 3rd with the upper note D#, whose details are shown in red because this is now a consonant interval
[2]. Remembering what was said previously, this means that both notes must always be assigned to separate channels, and the table shows that this is so. The subsequent entries across this row cover all of the other intervals sharing the same lower note (C) in the same way.
Moving to the second row enables us to analyse in a similar way all of the intervals whose lower note is this time C#, and again we see that all consonant intervals have independent channels assigned to their notes as required. Subsequent rows cover all other possible intervals except for the octaves, which always use the same channel for both notes as explained earlier.
There is a total of 132 intervals in the table, of which 72 are consonant and 60 dissonant. All consonant intervals (shown in red) are routed through different channels as required. Of the dissonant ones, the notes of 12 are routed through the same channel whereas 48 use different channels. Therefore, even though the number of channels has been halved in this scheme compared to the previous one, the only penalty is that 12 out of the 60 dissonant intervals now use the same channel.
Regarding the note-routing of triads, it has already been explained that each note of all 24 triads will be routed through different channels provided this happens for all the consonant intervals. This can easily be proved by picking off from the table the channels corresponding to the notes of each triad, and the results are shown in Table 2.
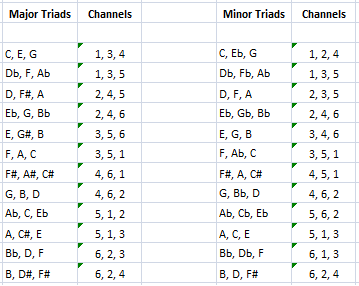
Table 2. Note distributions for major and minor triads - 6 channel system
In summary, a 6-channel system offers nearly all the advantages of a fully separated 12-channel one in terms of reducing IM distortion : all consonant intervals and all major and minor triads are routed separately, and of the 60 dissonant intervals only 12 use the same channel. IM distortion will arise for these 12 intervals only, but since they are dissonant it is doubtful whether it will be subjectively detectable. This is a modest price to pay for the considerable economies gained through halving the number of amplifiers and loudspeakers.
4-channel system
In this system, groups of three adjacent notes now share a common audio channel. Thus C, C# and D could be one group; D#, E and F the next and so on. Four such groups will therefore cover an octave, reducing the number of amplifiers and loudspeakers still further. As before, the whole instrument could use a single array of four channels, or there might be separate 4-channel arrays for each division, for small groups of stops or even for individual stops. The more elaborate realisations would also address the problem of phase interference due to electronic rather than acoustic signal mixing.
In this scheme it should be clear that certain intervals, comprising not just semitones but whole tones as well (though not all of them), are each handled by a single audio channel. The justification for this was discussed earlier. These are among the most dissonant intervals, and their subjective coarseness should assist in masking intermodulation distortion in the channel. However it is necessary to analyse in more detail the assignment of intervals to audio channels before drawing further conclusions. Let us therefore look at a system with the following channel assignments:
Channel 1: C, C#, D
Channel 2: D#, E, F
Channel 3: F#, G, G#
Channel 4: A, A#, B
The way that the various notes and intervals are distributed across the four audio channels is shown in Table
3 below:
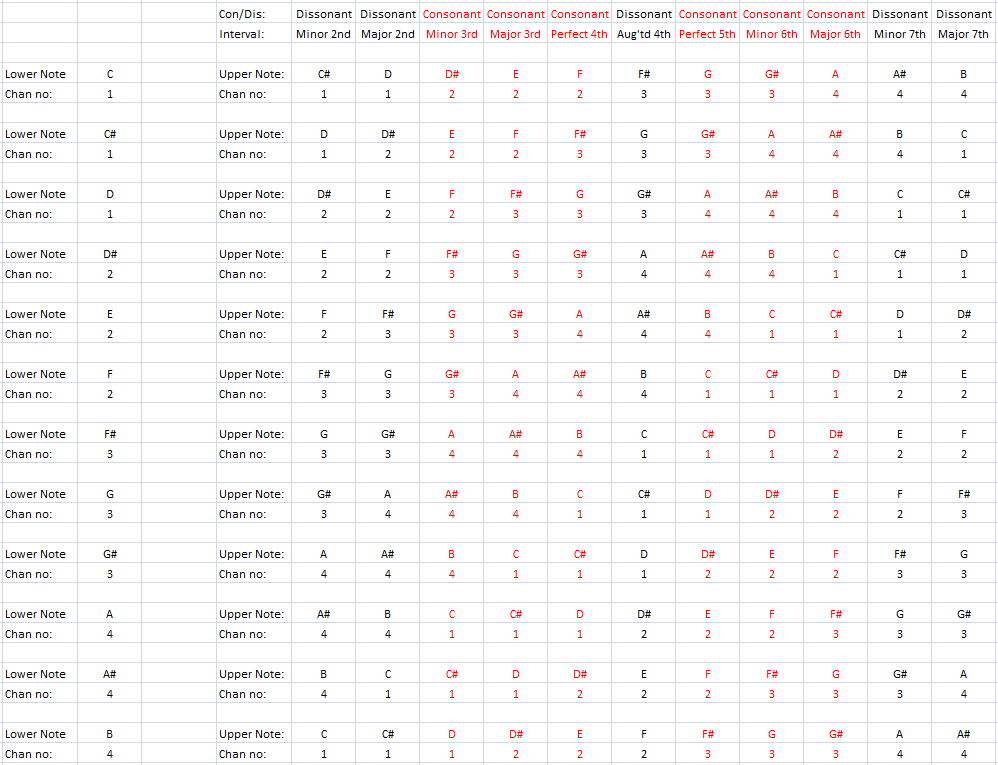
Table 3. Distribution of notes and intervals to audio channels - 4 channel system
This table has the same format as the previous one for the 6-channel system so hopefully no further explanation will be needed this time. In fact the two tables are identical except for the channel numbers printed underneath each note.
In terms of statistics, there is a total of 132 intervals in the table, of which 72 are consonant and 60 dissonant. These numbers remain the same as previously because they have no connection with the number of independent audio channels. All consonant intervals (shown in red) are routed through different channels, as required. However, this time the notes of 24 dissonant intervals are now routed through the same channel rather than 12 in the 6-channel system. The remaining 36 use different channels. Therefore, even though the number of channels has been reduced still further in this scheme, the majority of dissonant intervals still speak through separate channels.
Regarding the note-routing of triads, it has already been explained that each note of all 24 triads will be routed through different channels provided this happens for all the consonant intervals. This can easily be proved by picking off from the table the channels corresponding to the notes of each triad, and the results are shown in Table 4.

Table 4. Note distributions for major and minor triads - 4 channel system
In summary, a 4-channel system still offers nearly all the advantages of a fully separated 12-channel one in terms of reducing IM distortion: all consonant intervals and all major and minor triads are routed separately, and of the 60 dissonant intervals only 24 use the same channel. IM distortion will arise for these 24 intervals only, but since they are dissonant it is doubtful whether it will be detectable. This is a modest price to pay for the enormous economies gained by using only one third the number of amplifiers and loudspeakers.
It is not possible to reduce the number of independent audio channels beyond this because IM distortion will creep in with certain consonant intervals and triads. For example, bracketing four adjacent notes such as C, C#, D and D# means that the notes of a consonant minor third
(C-D#/E♭) would share the same channel. This is contrary to the rule established at the outset which demands that all 72 consonant intervals and all 24 triads speak through separate channels.
Concluding remarks
A well known shortcoming of digital organs is their tendency to fatigue the ear
rapidly when played loudly with many stops drawn. This commonly happens even in instruments which sound well when only one or two quiet stops are used. The phenomenon is
partly due to intermodulation distortion arising mainly in the loudspeakers which causes very large numbers of spurious sum and difference tones to be added to the sound. It is quite possible for there to be hundreds of thousands of distortion products, and although each is of low amplitude, their sheer number causes an audible background of acoustic mush to arise in the radiated sound. As a result the sound loses transparency and it becomes wearisome and identifiably electronic. Pipe organs do not suffer from this defect at all.
One way to eliminate the problem is to use one or more banks of twelve independent audio channels, each channel handling only one note and its octaves. There is then no opportunity for intermodulation to occur. However the number of amplifiers and loudspeakers required can become excessive when several such banks are required, and this article has shown how it can be reduced up to threefold using banks of no more than four channels. This simplification is possible because it is unnecessary to use separate channels for the notes comprising dissonant intervals. Their subjective coarseness in effect masks the distortion products which they generate.
This approach cannot be applied to the purer-sounding consonant intervals however, which must therefore retain separate channels for their respective notes. This is essential if all the major and minor triads are also to be radiated without intermodulation distortion, because each triad is built from consonant intervals. As triads form the foundation of all harmony, it is important to handle them properly in a low-distortion channel assignment scheme.
The article has shown how to assign notes to banks of six and four audio channels to enable these principles to be applied. Either configuration represents a considerable saving in terms of hardware and cost over that of a twelve-channel system.
Notes and References
1. The End of the Pipe
Organ?, an article on this website, C E Pykett 2005.
Material relevant to this article is in the 'Technical Matters' section.
2. The implication in the text that the interval C-D# is a minor third is musical nonsense, because it should properly be written as
C-E♭ even though the notes played on the keyboard are the same. The anomaly arises because
Table 1 uses the tuner's convention of calling notes 'naturals' and 'sharps' regardless of scale or key. It has been done for convenience and it is assumed readers will understand and accept it.